Hockey sticks on planet ABBABA

Question: On the planet ABBABA, the inhabitants have a binary language where the only two letters in their alphabet are A and B. The language is incredibly efficient and complex in that every finite sequence of A’s and B’s is a valid word. How many of the words in this language have exactly five A’s and at most five B’s?

For instance, ABAAABA, AAAAA, and BBBBBAAAAA are all valid such words, since they all have five A’s and no more than five B’s.

The CW complex structure of the Grassmannian

In a previous post, we briefly described the complex Grassmannian $\mathrm{Gr}(n,k)$ as a CW complex whose cells are the Schubert cells with respect to a chosen flag. We’ll now take a closer look at the details of this construction, along the lines of the exposition in this master’s thesis of Tuomas Tajakka (chapter 3) or Hatcher’s book Vector Bundles and $K$-theory (page 31), but with the aid of concrete examples.

Background on CW complexes

Let’s first review the notion of a CW complex (or cell complex), as described in Hatcher’s Algebraic Topology.

An $n$-cell is any topological space homeomorphic to the open ball $B_n=\{v\in\mathbb{R}^n:|v|<1\}$ in $\mathbb{R}^n$. Similarly an $n$-disk is a copy of the closure $\overline{B_n}=\{v\in \mathbb{R}^n:|v|\le 1\}$.

To construct a cell complex, one starts with a set of points called the $0$-skeleton $X^0$, and then attaches $1$-disks $D$ via continuous boundary maps from the boundary $\partial D$ (which simply consists of two points) to $X^0$. The result is a $1$-skeleton $X^1$, which essentially looks like a graph:

Higher specht polynomials

There are polynomials. There are Specht polynomials. And then there are higher Specht polynomials.

A colleague recently pointed out to me the results of this paper by Ariki, Terasoma, and Yamada, or more concisely summarized here. The authors give a basis of the ring of coinvariants \[R_n=\mathbb{C}[x_1,\ldots,x_n]/(e_1,\ldots,e_n)\] which, unlike the other bases we’ve discussed on this blog so far, respects the decomposition into irreducible $S_n$-modules. These basis elements include the ordinary Specht polynomials, but also some polynomials of higher degrees, hence the name ``higher’’ Specht polynomials.

Background: What is a Specht polynomial?

The construction of the irreducible representations of the symmetric group $S_n$ (called Specht modules) is often described in terms of ``polytabloids’’, but can be equivalently described in terms of a basis of Specht polynomials.

Schubert Calculus mini-course

I’ve written a lot about Schubert calculus here over the last few years, in posts such as Schubert Calculus, What do Schubert Curves, Young tableaux, and K-theory have in common? (Part II) and (Part III), and Shifted partitions and the Orthogonal Grassmannian.

Pythagorean triples on a sphere?

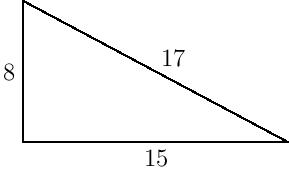
It’s 8/15/17, which means it’s time to celebrate! The three numbers making up the date today form a Pythagorean triple, a triple of positive integers $(a,b,c)$ with $a^2+b^2=c^2$. Indeed, $8^2+15^2=64+225=289=17^2$.

Alternatively, by the Pythagorean theorem, a Pythagorean triple is any triple of positive integers which make up the sides of a right triangle:

It’s exciting when all three sides are integers, since many common right triangles’ lengths involve square roots: $(1,1,\sqrt{2})$, $(1,2,\sqrt{5})$, and $(1,\sqrt{3},2)$, to name a few. And these sides aren’t even rational, which the poor Pythagorean scholar Hippasus discovered by proving that $\sqrt{2}$ is irrational and was subsequently drowned to death by his colleagues, according to some historical accounts. So the ancient Pythagoreans in fact only believed in right triangles having rational side lengths.